Topic 5 Applications of Integrals

5.1 Area between Curves

To find the area between curves, we identify a variable and use the differential in that variable as the width and the distance between the curve as the height and approximate the area using the Riemann sum of such a partition.

If the curves are given by $y=f\left(x\right)$ and $y=g\left(x\right)$ with $a\le x\le b$, then the area between the curves may be set up as $$\text{Area Between Curves}={\int }_a^b|f\left(x\right)-g\left(x\right)|dx$$ Because the distance in $y$ direction is easier to calculate.

If the curves are given by $x=p\left(y\right)$ and $x=q\left(y\right)$ with $c\le y\le d$, then the area between the curves may be set up as $$\text{Area Between Curves}={\int }_c^d|p\left(y\right)-q\left(y\right)|dy$$ Because the distance in $x$ direction is easier to calculate.

The absolute value sign can be removed by breaking the area into sub-areas.

Exercise 5.1 Sketch the graph of curves $y=4-x^2$, $y=2x+1$, $x=-2$, and $x=0$, and find the area enclosed by those curves.

Solution.

The graph of the curves shows that a vertical rectangular slice of the region has an area $\left[\right(4-x^2)-(2x+1\left)\right]dx$.

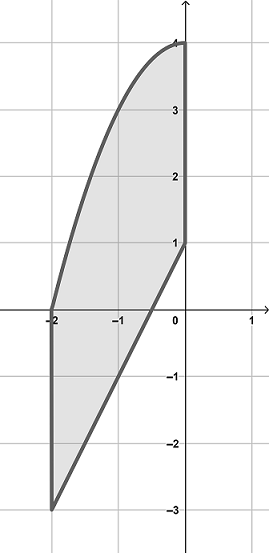
Area Between Two Curves

Then the area of the region can be calculated by the definite integral $${\int }_{-2}^0\left[\right(4-x^2)-(2x+1\left)\right]dx=(-\frac{x^3}{3}-x^2+3x){|}_{-2}^0=\frac{22}{3}.$$

Exercise 5.2 Sketch the graph of curves $y=x^2-2$ and $y=2$, and find the area enclosed by those curves.

Solution.

Solve the system of equations $$\{\begin{array}{c}y=x^2-2\\ y=2,\end{array}$$ we get two intersection points $(-2,2)$ and $(2,2)$. Over the interval $[-2,2]$, $x^2-2\le 2$. Then the area of the region can be calculated by the definite integral $${\int }_{-2}^2[2-(x^2-2\left)\right]dx=2{\int }_0^2(4-x^2)dx=2(4x-\frac{x^3}{3}){|}_0^2=\frac{32}{3}.$$

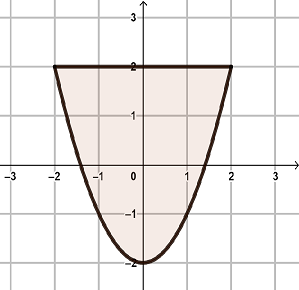
Area Between Two Curves

Exercise 5.3 Sketch the graph of curves $y=x^2+x-2$ and $y=1-x$, and find the area enclosed by those curves.

Solution.

Solve the system of equations $$\{\begin{array}{c}y=x^2+x-2\\ y=1-x,\end{array}$$ we get two intersection points $(-3,4)$ and $(1,0)$. Since the function $(x^2+x-2)\le (1-x)$ for $-3\le x\le 1$, the area of the region can be calculated by the definite integral $${\int }_{-3}^1\left[\right(1-x)-(x^2+x-2\left)\right]dx=(3x-\frac{x^2}{2}-\frac{x^3}{3}){|}_{-3}^1=\frac{32}{3}.$$

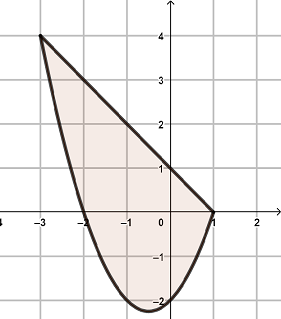
Area Between Two Curves

Exercise 5.4 Sketch the graph of curves $y=\cos x$ and $y=2\sin x\cos x$ with $-\pi /2\le x\le \pi /2$ and find the area between those curves.

Solution.

Solve the system of equations $$\{\begin{array}{c}y=\cos x\\ y=2\sin x\cos x,\end{array}$$ we get three intersection points $(-\pi /2,0)$, $(\pi /6,\sqrt{3}/2)$ and $(\pi /2,0)$. The graph of the functions are shown below.

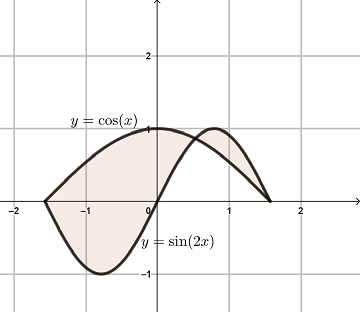
Area Between Two Curves

The area of the region can be calculated by the sum of two definite integral $$\begin{array}{cc}& {\int }_{-\pi /2}^{\pi /2}|\cos x-2\sin x\cos x|dx\\ =& {\int }_{-\pi /2}^{\pi /6}[\cos x-2\sin x\cos x]dx+{\int }_{\pi /6}^{\pi /2}[2\sin x\cos x-\cos x]dx\\ =& -\frac{1}{4}(1-2\sin x{)}^2{|}_{-\pi /2}^{\pi /6}+\frac{1}{4}(2\sin x-1){|}_{\pi /6}^{\pi /2}\\ =& \frac{9}{4}+\frac{1}{4}\\ =& \frac{5}{2}.\end{array}$$

Exercise 5.5 Sketch the graph of curves $y=|x|$ and $y=-x^2+2$ and find the area enclosed by those curves.

Solution.

Solve the system of equations $$\{\begin{array}{c}y=|x|\\ y=-x^2+2,\end{array}$$ we get three intersection points $(-1,1)$, and $(1,1)$. The graph of the functions are shown below.

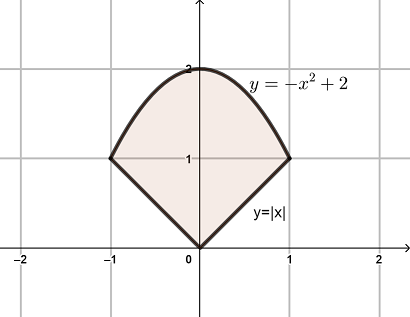
Area Between Two Curves

The area of the region can be calculated by the definite integral $$\begin{array}{cc}& {\int }_{-1}^1||x|-(-x^2+2)|dx\\ =& 2{\int }_0^1||x|-(-x^2+2)|dx\\ =& 2{\int }_0^1(-x^2+2-x)dx\\ =& 2(-\frac{x^3}{3}-\frac{x^2}{2}+2x){|}_0^1\\ =& \frac{7}{3}.\end{array}$$

Exercise 5.6 Sketch the graph of curves $x+y^2=5$ and $y^2=x+3$ and find the area enclosed by those curves.

Solution.

Solve the system of equations $$\{\begin{array}{c}x+y^2=5\\ y^2=x+3,\end{array}$$ we get three intersection points $(1,-2)$, and $(1,2)$. The graph of the functions are shown below.

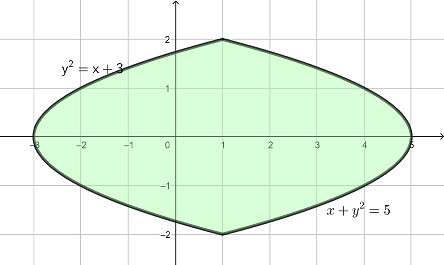
Area Between Two Curves

The area of the region can be calculated by the definite integral $$\begin{array}{cc}& {\int }_{-2}^2|(5-y^2)-(y^2-3)|dy\\ =& 2{\int }_0^2(-2y^2+8)dy\\ =& 2(-\frac{2y^3}{3}+8y){|}_0^2\\ =& \frac{64}{3}.\end{array}$$

Exercise 5.7 Sketch the graph of curves $y=x$ and $x=y^2-y-3$ and find the area enclosed by those curves.

Solution.

Solve the system of equations $$\{\begin{array}{c}y=x\\ x=y^2-y-3,\end{array}$$ we get three intersection points $(-1,-1)$, and $(3,3)$. The graph of the functions are shown below.

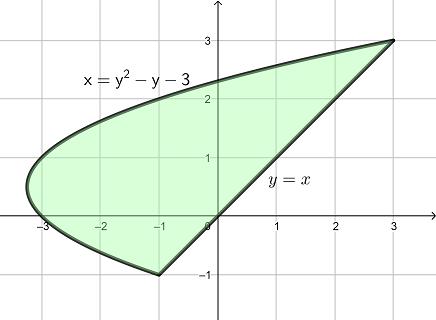
Area Between Two Curves

The area of the region can be calculated by the definite integral $$\begin{array}{cc}& {\int }_{-1}^3|y-(y^2-y-3)|dy\\ =& {\int }_{-1}^3(-y^2+2y+3)dy\\ =& (-\frac{y^3}{3}+y^2+3y){|}_{-1}^3\\ =& \frac{32}{3}.\end{array}$$

Exercise 5.8 Sketch the graph of curves $x=y^4$ and $y^2=2-x$ and find the area enclosed by those curves.

Solution.

Solve the system of equations $$\{\begin{array}{c}x=y^4\\ y^2=2-x,\end{array}$$ we get three intersection points $(1,-1)$, and $(1,1)$. The graph of the functions are shown below.

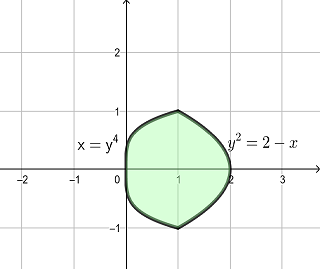
Area Between Two Curves

The area of the region can be calculated by the definite integral $$\begin{array}{cc}& {\int }_{-1}^1|y^4-(2-y^2)|dy\\ =& 2{\int }_0^1(2-y^2-y^4)dy\\ =& 2(-\frac{y^5}{5}-\frac{y^3}{3}+2y){|}_0^1\\ =& \frac{44}{15}.\end{array}$$

Exercise 5.9 Sketch the graph of curves $y=x^2-1$ and $y=x^3+x^2-x-1$ and find the area enclosed by those curves.

Solution.

Let $D\left(x\right)=(x^2-1)-(x^3+x^2-x-1)=-x^3+x$. Solve $D\left(x\right)=0$, we get three solutions $x=-1$, $x=0$, and $x=1$. Using test point method or algebraic method, we find that $D\left(x\right)<0$ for $-1<x<0$ and $D\left(x\right)>0$ for $0<x<1$. The graph of the functions are shown below.

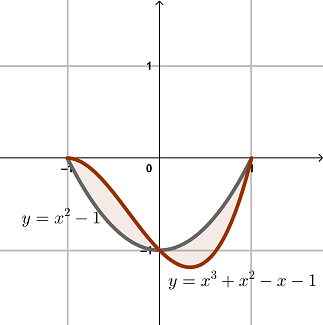
Area Between Two Curves

The area of the region can be calculated by the sum of two definite integral $$\begin{array}{ccc}& {\int }_{-1}^1|-x^3+x|dx& \\ =& 2{\int }_0^1(-x^3+x)dx=& 2(-\frac{x^4}{4}+\frac{x^2}{2}){|}_0^1\\ =& \frac{1}{2}.& \end{array}$$

Exercise 5.10 Sketch the graph of curves $x-2y=0$ and $y^3-y^2=x$ and find the area enclosed by those curves.

Solution.

Since $x$ is easier to solve, it is better to use the sum of areas horizontal rectangles, that is the area is given by $${\int }_a^b|x_R\left(y\right)-x_L\left(y\right)|dy.$$ Solve for $x$ from the equations of the curves and set $D\left(y\right)=(y^3-y^2)-2y=y^3-y^2-2y$. Solve $D\left(y\right)=0$, we get three solutions $y=-1$, $y=0$, and $y=2$. Using test point method or algebraic method, we find that $D\left(y\right)>0$ for $-1<y<0$ and $D\left(y\right)<0$ for $0<y<2$. The graph of the functions are shown below.

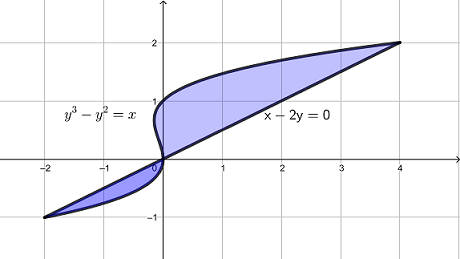
Area Between Two Curves

The area of the region can be calculated by the sum of two definite integral $$\begin{array}{cc}& {\int }_{-1}^2|y^3-y^2-2y|dy\\ =& {\int }_{-1}^0(y^3-y^2-2y)dy-{\int }_0^2(y^3-y^2-2y)dy\\ =& (\frac{y^4}{4}-\frac{x^3}{3}-y^2){|}_{-1}^0-(\frac{y^4}{4}-\frac{x^3}{3}-y^2){|}_0^2\\ =& \frac{5}{12}+\frac{8}{3}\\ =& \frac{37}{12}.\end{array}$$